%@P:exocorcp
%@Dif:2
\begin{myenumerate}
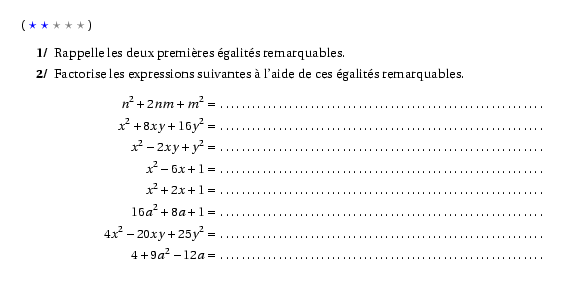
  \item Rappelle les deux premières égalités remarquables.
  \item Factorise les expressions suivantes à l'aide de ces égalités remarquables.
\[\Eqalign{
n^2+2nm+m^2&=\hbox to10cm{\dotfill}\cr
x^2+8xy+16y^2&=\hbox to10cm{\dotfill}\cr
x^2-2xy+y^2&=\hbox to10cm{\dotfill}\cr
x^2-6x+1&=\hbox to10cm{\dotfill}\cr
x^2+2x+1&=\hbox to10cm{\dotfill}\cr
16a^2+8a+1&=\hbox to10cm{\dotfill}\cr
4x^2-20xy+25y^2&=\hbox to10cm{\dotfill}\cr
4+9a^2-12a&=\hbox to10cm{\dotfill}\cr
}\]
\end{myenumerate}
%@Correction:
\[\Eqalign{
n^2+2nm+m^2&=(n+m)^2\cr
x^2+8xy+16y^2&=(x+4y)^2\cr
x^2-2xy+y^2&=(x-y)^2\cr
x^2-6x+9&=(x-3)^2\cr
x^2+2x+1&=(x+1)^2\cr
16a^2+8a+1&=(4a+1)^2\cr
4x^2-20xy+25y^2&=(2x-5y)^2\cr
4+9a^2-12a&=9a^2-12a+4=(3a-2)^2\cr
}\]